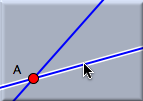
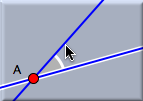
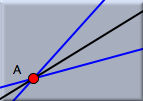
Angle Bisector

Angle Bisector

This mode is used to construct the angular bisector of two lines. The application of this mode requires a little care, since two lines do not have only one angular bisector; they have two! To take this fact into account, this mode is provided with a position-sensitive selection mechanism.

To define the angular bisector, two lines have to be selected. In order to indicate which angular bisector should be chosen, three points are relevant: the click point of the first selection, the click point of the second selection, and the intersection of the two selected lines. Imagine a triangle formed by these three points. The inner angle at the intersection point of the lines will be bisected. This is what you would intuitively expect.

To make the selection process a bit simpler, Cinderella gives graphical hints as to which angle will be bisected.

First selection: A line is highlighted. The selection point is memorized.		Moving the mouse: An indication of the chosen angle is given.		Second Selection: The angular bisector is added.